


Next: MHD equations
Up: Fluid & MHD Theory
Previous: Vlasov equations
In the fluid description, information
on the particle velocity distribution is replaced by values averaged
over velocity space.
The averaging is achieved by taking moments
of Boltzmann's equation (3.11).
We proceed by rewriting Boltzmann's equation in tensor form:
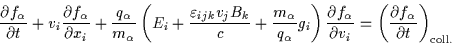 |
(3.18) |
Moment equations are obtained by multiplying (![[*]](l2hicons/gif/cross_ref_motif.gif) )
by an arbitrary function of velocity
)
by an arbitrary function of velocity
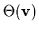 and integrating
over velocity space. The moment of the first term in (
and integrating
over velocity space. The moment of the first term in (![[*]](l2hicons/gif/cross_ref_motif.gif) )
is
)
is
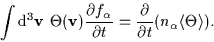 |
(3.19) |
Similarly the second term satisfies
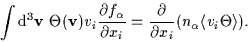 |
(3.20) |
After some algebra, the third term transforms to
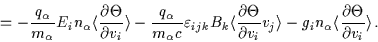 |
(3.21) |
The general moment equation has the form
 |
(3.22) |
Taking the zeroth order moment, with
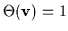 ,
(
,
(![[*]](l2hicons/gif/cross_ref_motif.gif) ) gives
) gives
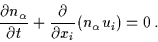 |
(3.23) |
Note that the right-hand side is zero due to particle conservation for
ions and electrons (for an ideal plasma, ignoring ionization,
recombination and charge exchange effects); i.e.,
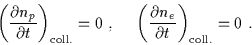 |
(3.24) |
Mass conservation equation:
multiply (![[*]](l2hicons/gif/cross_ref_motif.gif) ) by
) by
 and sum over
and sum over  :
:
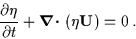 |
(3.25) |
Charge conservation equation:
Multiply (![[*]](l2hicons/gif/cross_ref_motif.gif) ) by
) by
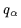 and sum over
and sum over  :
:
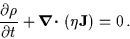 |
(3.26) |
We take the first order moment (
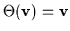 )
by
multiplying (
)
by
multiplying (![[*]](l2hicons/gif/cross_ref_motif.gif) ) by vs and integrating over velocity space,
) by vs and integrating over velocity space,
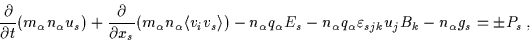 |
(3.27) |
where the sign of the momentum density
Ps is opposite for electrons and ions, and Psis defined by
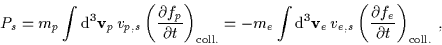 |
(3.28) |
since collisions between electrons and ions within the plasma
do not change the total
momentum density of the system.
Using the defined fluid quantities for ui, Ui, wi and pij,
and using
the relation:
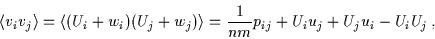 |
(3.29) |
equation (![[*]](l2hicons/gif/cross_ref_motif.gif) ) may be re-expressed in the form (omitting
particle species
) may be re-expressed in the form (omitting
particle species  ),
),
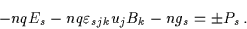 |
(3.30) |



Next: MHD equations
Up: Fluid & MHD Theory
Previous: Vlasov equations
Iver Cairns
1999-08-09
![]() ,
(
,
(![]() ) gives
) gives
![]() )
by
multiplying (
)
by
multiplying (![]() ) by vs and integrating over velocity space,
) by vs and integrating over velocity space,