Physics
of Baseball & Softball
** A new article on knuckleballs
was added in May 2013 **
The following material has been expanded considerably into a 324 page
book “Physics of baseball and softball” published by Springer in March 2011.
Alan Nathan has the world's best Physics of Baseball
site at http://go.illinois.edu/physicsofbaseball
Another very interesting Physics of Baseball and
Softball site is maintained by Dan Russell at http://www.kettering.edu/~drussell
Lloyd Smith at Washington State University has an
excellent site on the physics and engineering of softball, at http://www.mme.wsu.edu/~ssl
, including information on how bats and balls are tested and certified for use
in the USA.
James Sherwood has a similar site http://m-5.eng.uml.edu/umlbrc
describing test procedures used to certify baseball bats and balls at the
University of Massachusetts – Lowell.
1. The sweet spot
Batters know from experience that there is a sweet spot on the bat,
about 17 cm from the end of the barrel, where the shock of the impact, felt by
the hands, is reduced to such an extent that the batter is almost unaware of
the collision. At other impact points, the impact is usually felt as a sting or
jarring of the hands and forearm, particularly if the impact occurs at a point
well removed from the sweet spot.
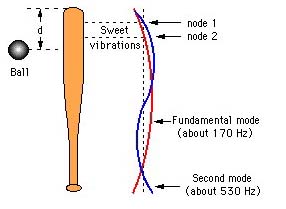
The sweet spot of a bat exists because bat vibrations
are not excited significantly at that spot. The spot is close to the centre of
percussion (COP) for a freely supported bat, so it was thought at one time that
the sweet spot might be associated with the COP. However, the COP shifts a long way toward the
handle when a batter holds the handle, and plays only a minor role in the feel
of the bat for an impact at the sweet spot.
These spots are also described in the physics of
tennis page. A bat is similar to a racquet but there are some obvious
differences. For a tennis racquet only the fundamental mode of vibration is
excited by the impact since the impact duration, about 5 msec, is too long to
excite higher frequency modes of the racquet. The impact on a baseball bat is
shorter (since the bat and ball are harder or stiffer), about 1 msec. As a
result, the fundamental and second vibration modes are both excited with about
the same amplitude. These modes are shown in the diagram above. Hence, there
are two vibration nodes in the barrel. An impact at the fundamental node will
not excite that mode, but it will excite the second mode. Similarly, an impact
at the node of the second mode will not excite the second mode but it will
excite the fundamental mode.
Most people find that an impact between the nodes of
the first two modes (node1 and node2 in the diagram) feels best since both
modes are then excited only weakly.
For further details, see American Journal of Physics,
September 1998 and also American Journal of Physics, 69,
231-232 (2001). The effect of the hands on the centre of percussion is
described in American Journal of Physics, 72, 622-630 (2004). Both papers can be downloaded here and here.
2. A Physics of Baseball Project
The experiment described below would be an ideal
project on the physics of baseball, for any high school or University physics
student. There is enough physics in it to keep a PhD student busy for a few
years, but it could also be done
by any high school student as a simple introduction to the physics of baseball.
The experiment can be done at low bat and ball speeds since the essential physics
of the problem does not depend on the actual bat and ball speeds. Rather, the
physics depends more on the ratio of the bat and ball speeds, regardless of
whether the actual speeds are around 1 mph or 100 mph. Some of the numbers
change with actual speed, but the physics doesn’t change. It is not quantum
physics or relativity, but a very interesting problem involving the mechanics
of colliding objects.
The idea is to mount a bat as a pendulum and strike it
with a ball at various spots along the barrel. One question that can be
answered this way is "Where on the bat does the ball bounce best?" In
theory, the ball bounces best for an impact at the center of mass of the bat,
provided we can ignore bat vibrations. But the bat vibrates strongly for
impacts near the tip of the bat and near its center of mass. Energy is lost
during the collision if the bat vibrates. As a result, the bounce is relatively
weak at the tip and near the center of mass.
If the ball is also mounted as a pendulum, the experiment can also be performed in
reverse, by swinging the bat at a stationary ball. We can then ask a similar
question. Where on the bat is the ball struck with the greatest speed? A first
guess would be at the tip of the bat since the tip travels the fastest. But
strong vibrations are generated for impacts near the tip. Furthermore, the tip
is the lightest and least effective part of the bat.
By combining the results of these two separate
experiments, we can then work out what happens when a bat is swung at an incoming
ball. The outgoing speed of the ball is just the sum of the two separate parts
- the speed when the bat is at rest plus the speed when the ball is at rest.
Some results for an Easton BK7 aluminum bat are shown
below in 13 QuickTime movie files, each about 1 Mb. Also shown for comparison
is a bounce off a heavy block of granite so that the COR of the ball can be
determined. For small swings of a pendulum, the incoming and outgoing speed of
the pendulum bob is proportional to its horizontal displacement, so the
relevant ball speed ratios can be measured directly off the film with a ruler.
The bat is 84 cm long, and has a mass of 849 gram. Its center of mass is 52.6
cm from the knob end. The bat was swung through an axis 143 mm from the knob
end. Its moment of inertia about
an axis through its center of mass is 0.184 kg.m^2. The impacts were at
distances of 2, 7, 12, 17, 24 and 31 cm from the end of the barrel.
Impact at 2 cm Impact at 7 cm Impact at 12 cm Impact at 17 cm
Impact at 24 cm Impact at 31 cm
Strike
at 2cm Strike at 7 cm
Strike at 12 cm Strike at 17 cm Strike at 24 cm Strike at 31 cm.
The lines behind the bat, passing through the axis,
mark out angles of 5, 10, 15, 20, 25 and 30 degrees. The bat was swung through
an angle of about 30 degrees to strike the ball. Each impact was recorded on a
video camera at 25 frames/sec.
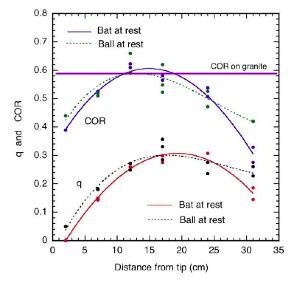
Coefficient of Restitution = Relative speed of bat and
ball after collision/ relative speed before collision, where "speed of
bat" is the speed of the bat at the impact point.
The COR for the impact on the granite block is about
0.59 in the low speed collision here, but can be as low as 0.45 in a 100 mph
collision. The COR for a collision between the bat and the ball is less than
0.59 when energy is lost to vibrations, but can be greater than 0.59 if the bat
has a trampoline effect on the ball. A high performance bat has a strong
trampoline effect and hence a relatively large COR. The technique here can be be used to measure the performance
of any bat, at least at low ball speeds. The swing weight of the bat (that is, its moment of inertia) can be
determined from the measured period of oscillation when swung as a pendulum.
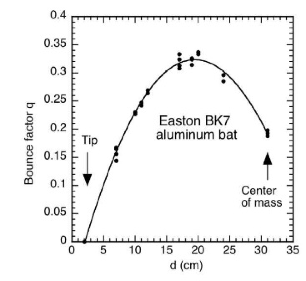
The bounce factor found from these and some additional
impacts is shown below.
The relevant collision equations are derived in the
Physics of Baseball and Softball book (and elsewhere) and are:
1. If the
bat is at rest then we can define the ball speed ratio
Bounce Factor = q = (ball exit speed) / (incident ball
speed)
q is also called the apparent coefficient of
restitution or ACOR. q varies from about zero near the tip of the bat to about
0.2 or 0.3 further along the barrel, and needs to be measured for any given bat
since it is usually too hard to calculate. So,
Ball exit speed = q*vin where vin =
incident ball speed.
2. If the ball is at rest (when striking a ball off a
T ) then
Ball exit speed = (1 + q)V where V = bat speed at impact
point just before the impact.
3. If the bat approaches a ball incident at speed vin
then
Ball exit speed = (1 + q)V + q*vin
The impact point 2 cm from the tip of the Easton bat
is a dead spot where the ball doesn't bounce at all, so q = 0. However, when
the bat strikes the ball at the dead spot then the ball exit speed = V = speed
of bat just before the collision.
3. Swing speed vs bat weight
Are heavy bats are more powerful than light bats? The answer
depends on how fast each bat can be swung. The following three movies show a
batter swinging three different bats as fast as possible, filmed at 25
frames/sec. One was a 232 gram
broomstick, one was a 871 gram Louisville Slugger wood bat with four holes
drilled through the barrel, and one was the 871 gram bat increased to 1333 gram
by inserting a steel bolt through each hole. The 232 g bat was swung at 45
radians/sec on average (by the batter in the film). The same batter swung the
871 g bat at 33 radians/sec on average, and he swung the 1333 g bat at 26
radians/sec on average. If you turn the volume up you will hear how the swing
speed affects the sound of the “swish” through the air.
232 g bat 871 g bat 1333
g bat
The above results are more or less as expected, in
that light bats can be swung faster than heavy bats. However, there are many variables in experiments
like these. It is not the actual weight that determines the swing speed but the
swing weight (ie the moment of inertia). In general, swing weight increases
with actual weight, but swing
weight also depends on bat length and weight distribution. It has been found
that the swing speed for any given batter is proportional to 1/(Swing
weight)^n where n is typically
about 0.27 for most batters. A
similar result is obtained when swinging cylindrical rods, as described in a
paper that can be downloaded here.
The weight and construction of a bat also affects the
bounce factor q, and it affects the time and position of the bat at which the
swing speed is a maximum. The batter might find that he needs to change the way
he swings the bat if the swing weight is altered, or he might have a “grooved”
swing technique and swing every bat the same way. There is no simple physics
answer to the heavy/light bat question here. In practice, batters tend to
prefer bats that are lighter, rather than more powerful, since lighter bats are
easier to swing and make it easier for the batter to connect with the ball.
A question that is equally interesting is how the
swing speed of each body segment varies as the bat speed varies and as the bat swing
weight varies. The shoulders reach maximum speed first, then the upper arms, then the forearm
and finally the bat. Any two connected segments can be analysed as a double
pendulum. The bat and the forearms are locked at right angles at the beginning of
the swing, by locking the wrists, otherwise the bat would start to rotate in
the wrong direction at the beginning of the swing. Near the end of the swing,
the bat swings so fast that the batter can no longer keep his wrists locked,
and the centripital force on the bat is about equal to the weight of the
batter.
4. How to swing a bat
It is not difficult to swing a bat. It is almost as
easy as walking. But how does a
batter do it? Specifically, what forces and torques are exerted on the handle,
and in what directions do they act?
It is very surprising that no one seems to have worked this out before.
Adair provides a few answers in his book “The Physics of Baseball” but he does not give the directions or
the torques. The diagram below shows the swing of a wood bat filmed from a spot
above the batter’s head. The force on the bat can be worked out from the
velocity of the center of mass, (CM), and the torques can be worked out from
the angular acceleration. The results are very surprising. Initially, the force
on the handle is in the opposite direction to the motion of the handle. While
the center of mass moves one way (nearly upward here), the handle moves the
opposite way (nearly downward). The batter needs to exert a small couple to get
the swing started, using equal and opposite forces on the handle, otherwise the
barrel of the bat will get left behind.
Near the end of the swing, the force is roughly at right angles to
motion of the handle since the centripetal force is very large. However, the
centripetal force does not act along the axis of the bat, but at an angle, as
shown by the orange lines.
The direction of the centripetal force is toward the
center of the circle followed by the path of the CM. Since the CM traces out a spiral rather than a circular
path, the center of the circle moves, as the bat is swung, along the path traced out by the inner
circle of black dots. At any given time, the center of the circle can be found
by fitting a circle to three neighboring points, at say time t, and at times t
+ 0.02 s and t - 0.02 s. This gives the radius, R, of the circle, from which we
can calculate the centripetal force MV2/R as well as the force at right angles to that, given
by M (dV/dt).
Near the end of the swing, the batter needs to exert a
large negative couple on the bat, otherwise the bat will swing around too fast
and strike the ball when it is aligned at the wrong angle. The same thing
happens when swinging a golf club, but it is not a well-known effect. Rather,
most coaches and others think in terms of wrist torque, which is probably much
too small to provide the necessary large couple near the end of the swing The
couple must come mainly from the two arms, not the wrists. A paper on this
subject can be downloaded here.

5. Bounce of a baseball
The impact of a bat and a ball is still not properly
understood. A lot has been learned over the years but more experiments need to
be done to better understand how the ball bounces and how much spin it acquires
when it bounces. The flight of a
ball through the air depends strongly on how fast it spins, and in what
direction it spins.
QuickTime movie files A and B show a baseball bouncing on a block of granite. The
bounce was filmed at 300 fps and is played back at 30 fps in slow motion. The
ball is 72 mm in diameter. From that you can work out the speed and spin of the
ball before and after it bounces. In principle you could also work out the
acceleration due to gravity in Sydney, but not very accurately.
The ball is spinning clockwise before it bounces and
hits the granite block with backspin.
The ball bounces backward spinning counter-clockwise. The reversal of
the spin in each bounce implies that there is some tangential elastic behaviour
of the ball in a direction parallel to the block. There is obviously some
elastic behaviour in the vertical direction as well, which is why the ball
bounces up off the block. Elastic behaviour in the vertical direction is
described by a number called the coefficient of restitution (COR) which is
about 0.5 for a baseball, depending on ball speed. If the ball didn't bounce at all then COR would be zero. If
there was no loss of energy during
the bounce then COR would be 1.0
Elastic behaviour in the horizontal direction can be
described by a tangential coefficient of restitution, which is about 0.5 or 0.6
for a superball but only about 0.2 for a baseball on a rigid surface. The tangential COR is defined in the
same way as the normal COR, but in terms of the horizontal speed of the ball at
the contact point rather than the normal (ie vertical) speed of the contact
point. The horizontal speed at the contact point depends on the rate at which
the ball spins, as well as on the horizontal speed of the centre of mass of the
ball. In file A, the ball spins faster than in file B, both before and after
the bounce. The ball seems to spin faster when it is fresh and slower after it
has been used, but more experiments are needed to determine whether this is in
fact correct. In file A, the tangential COR was surprisingly high, about 0.3.
Interesting information on the behaviour of a ball can
be obtained by bouncing it on a force plate or on a piezo to measure force vs
time. Typical results are shown here for a superball, a baseball and a
sorbothane ball. A superball has a COR of about 0.9, a baseball has a COR of
about 0.5 and a sorbothane ball has a COR of about 0.1 (ie it hardly bounces at
all). The shapes of the force vs time curves are interesting since they tell us
how the force on the ball varies with the amount of compression. Since F =
m*dv/t, we can integrate the force waveform to measure ball speed vs time, then
integrate v = dx/dt to measure the shift in the ball CM vs time.
 Superball, 0.5 ms/div
Superball, 0.5 ms/div
 Baseball, 0.2 ms/div
Baseball, 0.2 ms/div
 Sorbothane ball 2 ms/div
Sorbothane ball 2 ms/div
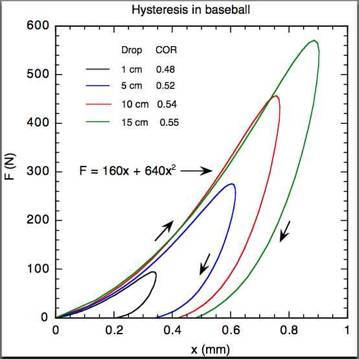
Graphs of F vs t and x vs t can also be used to
generate graphs of F vs x, showing how the force on the ball varies with its
compression. Results for a baseball dropped from heights of 1, 5, 10 and 15 cm
onto a piezo are shown below. The ball bounces while it is still compressed,
and recovers to its original sherical shape after it bounces. Similar results
are obtained at much higher ball speeds, the forces and x values being
correspondingly much larger. Balls compress along the same F vs x curve,
regardless of the ball speed, although the actual compression curve for a given
ball will depend on its stiffness.
6.
Bat and ball collisions
The collision of a bat and a ball can be understood by
treating the bat as a heavy ball whose mass depends on the impact point. The
whole mass of the bat is not involved in the collision, unless the collision
happens to occur at the centre of mass of the bat. Otherwise, the effective
mass of the bat is less than its whole mass. To understand such collisions it
is important to first understand the physics of a collision between one ball
and another. Movies showing the
collision of a baseball with a cricket ball and the collision of two billiard
balls are shown in the page on collisions.
A simple introduction to the physics of the collision problem
is given here.
7. Hoop Modes
A hollow aluminum bat sounds a lot different to a
solid bat since the wall of a hollow bat can vibrate in and out like a drum. Not
exactly like a drum, more like a bell or a chime, but the effect is similar.
The sound made by a bat can be described as a "ping". The frequency
is typically between 1000 Hz and 2500 Hz, meaning that the wall vibrates in and
out 1000 to 2500 times in one second. The end result can be a greater ball
speed off the bat, in the same way that a tennis ball bounces a lot better off
tennis strings than off a hard floor. The effect is known as the
"trampoline effect". There is a difference between a bat and a racquet
here, in that tennis strings are very light and give back to the ball 95% of
their stored elastic energy. The wall of a bat is relatively heavy and returns
only some of its stored elastic energy to the ball, keeping the rest to itself
so it can ping as loudly as it wants to. That is, a large fraction of the
stored elastic energy in the wall is retained as vibrational energy in the bat.
If the vibration frequency is less than about 1000 Hz, the end result is that
ball comes off the bat at a lower speed than off a wood bat of the same weight
and swing weight. For that reason, the walls of a hollow bat need to be
relatively stiff so they can vibrate at a frequency larger than 1000 Hz. Thick
walls are also needed to help prevent the walls dinting or cracking.
Some hoop mode sounds are recorded in the following
QuickTime Movies, using an aluminum bat and several aluminum tubes of various
diameters and wall thickness. The tubes were suspended by a length of string so
that they could vibrate for a long time without damping. It was found, by
measuring the frequencies of lots of aluminum tubes (see photo) that the frequency is directly
proportional to the wall thickness and inversely proportional to the tube
diameter squared. The stiffness of a cylinder was found to be roughly
proportional to its length x (wall thickness / diameter) squared (rather than
cubed, since the wall thickness is not negligible compared with the radius for
most of these tubes).
Cylinder 2 (500
Hz) Cylinder 3
(200 Hz)
Compare sounds here
(recorded with a signal generator connected to a speaker)

8. Softball spin speed
It is not easy to find information on the actual rate
of spin of a softball in flight, but it is relatively easy to measure, at least
at low ball speeds and low spin speeds. I tossed a 12 inch softball at very low
speed and filmed the result, using two hands instead of one to increase the
spin rate and to make sure the spin axis was horizontal. The result was not a
super slow softball spin, neither was it particularly fast. I simply wanted to
know whether a hand launched ball spins at around 100 rpm or whether it could
be as high as 1000 rpm. The ball rotated more than 1/4 but less than 1/2 of one
revolution from one frame to the next, each frame being 0.04 sec apart. Taking
the rotation rate as 3/8 revolution / 0.04 sec gives 9.4 revolutions / sec or
560 rpm. It would therefore not be surprising if a softball can be pitched at
say 1000 rpm. A rough estimate would be that the ball rotates 1/4 revolution
while it is in the pitcher’s hand,
and while the hand moves forward say the last 0.2 m. If the
hand moves forward at 10 m/s =
22.4 mph then it moves 0.2 m in 0.02 sec, so the spin rate would be 12.5
rev/s or 750 rpm. The hand moves forward faster than this in practice, so the
spin rate could easily be around 1500 rpm.
Another simple estimate of spin rate is to throw the
ball and catch it with the other hand as soon as it is released. The ball will
rotate about 1/2 a revolution from the initial grip to the release point, in
about 0.1 sec or less.
The film is shown here,
taken at 25 frames/sec.